
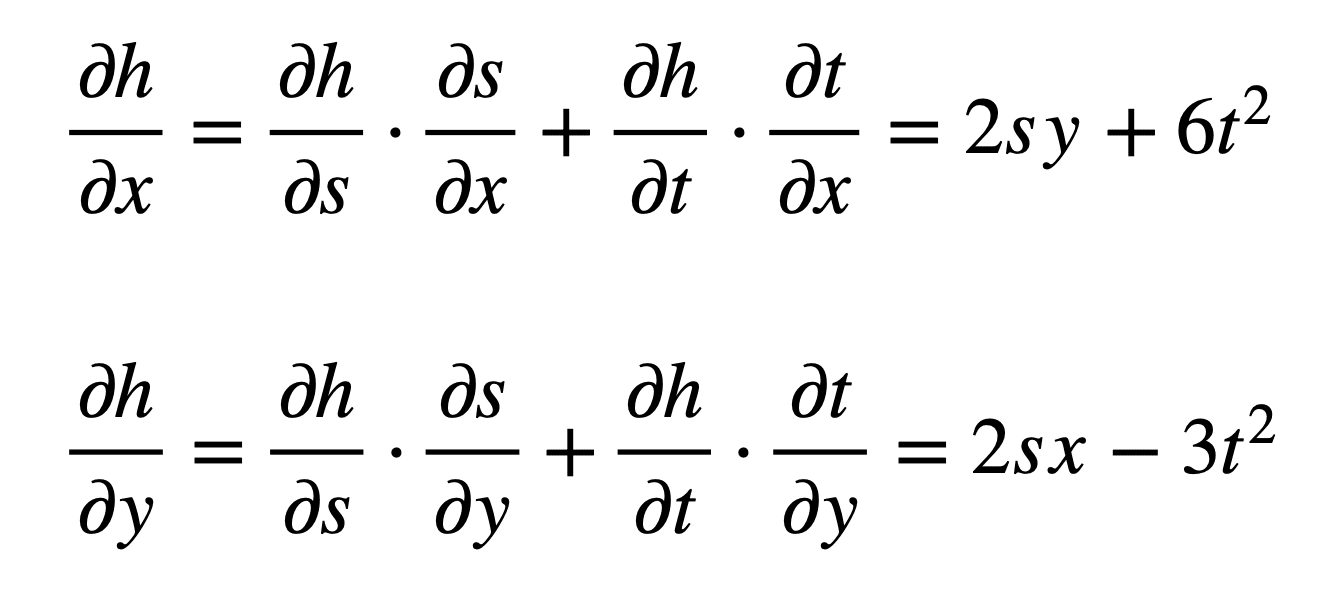
In most cases, final answers are given in the most simplified form.Ĭlick HERE to see a detailed solution to problem 1.Ĭlick HERE to see a detailed solution to problem 2.Ĭlick HERE to see a detailed solution to problem 3.Ĭlick HERE to see a detailed solution to problem 4.Ĭlick HERE to see a detailed solution to problem 5.Ĭlick HERE to see a detailed solution to problem 6.Ĭlick HERE to see a detailed solution to problem 7.Ĭlick HERE to see a detailed solution to problem 8.Ĭlick HERE to see a detailed solution to problem 9.Ĭlick HERE to see a detailed solution to problem 10.Ĭlick HERE to see a detailed solution to problem 11. This process will become clearer as you do the problems. (the term f'( g( x) ) ), then differentiate the inner layer (the term g'( x) ). Function f is the ``outer layer'' and function g is the ``inner layer.'' Thus, the chain rule tells us to first differentiate the outer layer, leaving the inner layer unchanged

For example, it is sometimes easier to think of the functions f and g as ``layers'' of a problem. Instead, we invoke an intuitive approach. However, we rarely use this formal approach when applying the chain rule to specific problems. In the following discussion and solutions the derivative of a function h( x) will be denoted by or h'( x). The chain rule is a rule for differentiating compositions of functions. The following problems require the use of the chain rule.


 0 kommentar(er)
0 kommentar(er)
